Practical Aspects of Mineral Thermobarometry

Part 1. Electron Microprobe Analysis

In an electron microprobe the chosen spot on the specimen is bombarded by a narrow beam of electrons, exciting secondary X-rays which are characteristic of the elements present in the sample. The characteristic X-ray spectrum for each element consists of a small number of specific wavelengths.

The essential parts of an electron microprobe:

Electron gun and a system of electromagnetic lenses for producing a focussed electron beam. Also scanning coils, to allow the beam to raster across an area of the specimen.
Specimen stage with x-y-z movement (many microprobes also have a light microscope for viewing the specimen)
Detection system. One or more solid-state detectors near the specimen, and/or wavelength spectrometers, with analysing crystal and detector(s)

There are two methods of detecting and quantifying the spectrum of secondary X-rays emitted from the specimen.

WDS: by wavelength, using a diffracting crystal to isolate the characteristic X-ray peaks.
EDS: by energy using a solid-state detector that discriminates between the energies of incoming photons.

Wavelength-dispersive spectrometry	Energy-dispersive spectrometry
High spectral resolution (elements clearly resolved) Higher peak/background = better detection limits Sequential measurement of peaks, slower Specimen must be flat and in focus Delicate moving parts Expensive to buy	Low spectral resolution (deconvolute overlapping peaks) Lower peak/background = lower precision Simultaneous collection of whole spectrum, rapid Insensitive to specimen geometry No moving parts Less expensive

Peaks and backgrounds (WDS)

The characteristic X-ray spectrum is superimposed on a background of "white" radiation in which all wavelengths are represented. The background, measured at a convenient offset from the peak position, is subtracted from the peak height. The background position must also be chosen so as not to overlap the peak for some other element which may occur in the specimen. The background is not always flat - in some cases it is important to measure background on both sides of the peak and interpolate the true background immediately under the peak.

Samples and standards

The net intensity of the characteristic X-ray peak is proportional to the mass concentration of that element in the specimen. However, quantitative analysis relies on comparing the specimen with a standard of known concentration of the element. The standards are calibrated in a separate session to establish the net counts per unit concentration for each element. The standards are natural or synthetic materials with accurately known composition, either because they are pure stoichiometric compounds, or because they have been analysed carefully by other techniques.

Correction procedures

The ratio of peak intensities measured on the specimen and standard gives an apparent concentration
C'_sp = C_std.(I_sp/I_std).
However, the intensities are affected by the difference in the nature and composition of the bulk material in the specimen and standard, so corrections of various kinds have to be applied. The most widely applied correction procedure is called ZAF, where the letters stand for and take into account:

Z - atomic number, which affects the penetration of incident electrons into the material
A - absorption of X-rays in the specimen, on the path to the detector
F - fluorescence caused by other X-rays generated in the specimen

The corrections are themselves a function of sample composition (which is what you're trying to determine!) so the correction procedure involves iteration, starting with estimated correction factors, until the input composition and the corrected composition converge.

Uncertainties and detection limits

Long, in Potts et al. (1995), page 18, gives the general formulae for determining the uncertainty on a measurement in the usual case where the important steps are subtraction of a background from a peak, and ratioing of two net peak counts for specimen and standard.

Other formulae for detection limits and uncertainties are at http://epmalab.uoregon.edu/UCB_EPMA/detectionlimits.htm

Analytical uncertainty

X-ray counts arise from random events, so the standard deviation () of a set of counts can be approximated by the square root of the total number of counts. Then:

For a sum or difference, 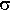= {(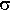₁)² + (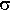₂)²}
For a ratio or product, 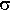/m = {(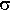₁/m₁)² + (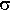₂/m₂)²}

where m is the mean or measured value. So, if the total counts on the peak and background are N_p and N_b respectively (peak and background being counted for the same length of time),
_{net peak} = (N_p + N_b)
and the overall uncertainty on the concentration, determined from the specimen-standard ratio, will be
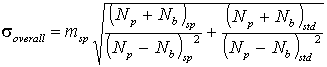
equation for uncertainty
For many elements that are present in moderate amounts, compared to a high concentration in the standard, the standard term will be comparatively small and the analytical uncertainty will be approximately given by
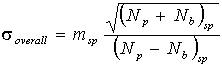
simlified equation for uncertainty

Typical values for the relative error /m are 0.3 to 1% on this basis for oxide concentrations between 50 and 2 weight %. In practice, the reproducibility will not be as good as this, as other instrumental factors come into play. For example, the theoretical 2 uncertainty on an analysis total, with a typical WDS configuration and 20 sec counting time per element, is ± 0.6 wt%. In a recent study (Waters & Charnley, 2002, Am. Min. 87) the actual 2 for the analysis totals of a population of 70 Ti-rich biotites analysed in a single session on one specimen was ± 1.34 wt%.

Detection limits

The detection limit is usually taken as a concentration equivalent to three standard deviations of the background counts, corresponding to a 99% probability that a peak significantly different from the background has actually been measured.
If the count rate and counting time on the background are I_b and t_b respectively, _b = ( I_b.t_b)

To convert this to concentration, we need the count rate per weight percent on the standard I_std, and the ZAF correction factor (approximately = 1). Then

Detection limit (wt %) = 3 . (ZAF) . 1/I_std .( I_b/t_b)

Detection limits for the routine analysis of common elements from Na to Fe are around 0.02 weight % (200 ppm).

Tips for acquiring good analyses

Calibrate carefully, and use one or more secondary standards as a check on the calibration.
Interpret as you analyse, don't wait and discover you've collected rubbish. Check the analytical totals (weight percent) and the the stoichiometry of the mineral formula (see Part 2).
Make good use of back-scattered electron imaging or line scans to detect intergrowths, compositional zoning and other features before you start quatntitative analysis.
Watch out for specimen damage. A focussed beam can damage certain minerals, such as feldspar and mica, leading to changing count rates and poor analysis totals. Most noticeable is usually a decrease in counts on alkali elements. Defocus the beam, or raster over a small area.

Sources and further reading

Pott, P. J. et al., (Eds.) 1995. Microprobe techniques in the Earth Sciences. Mineralogical Society Series 6, Chapman and Hall. Chapter 2 is specifically about the electron microprobe, but chapter 1 is worth reading as a general introduction to microbeam methods, and other chapters may be of interest.

Zussman, J. (Ed.) 1977. Physical Methods in Determinative Mineralogy, 2nd Ed.. Academic Press. Contains chapters on X-ray Fluorescence Spetrography (which includes the production of X-rays) and Electron Probe Microanalysis.

Smith D.G. (1976) Short Course in Microbeam Techniques. Mineralogical Association of Canada.

Some WWW resources

University of Oregon Electron Microprobe facility
has useful backgound information about the SEM and EPMA techniques

Yale University Electron Microprobe Laboratory

John Fournelle, University of Wisconsin-Madison:
Basic Information about electron microprobe analysis (EMPA)
Electron Microprobe Analysis course home page

University of Massachusetts Microprobe Lab
has an archive of spectacular compositional X-ray maps, with explanations.

Berkeley, Electron Microprobe Laboratory
Useful technical information, e.g. on calculating detection limits
Link is now broken, but pages appear to be reproduced at http://epmalab.uoregon.edu/UCB_EPMA/index.htm

^ Top

This page last modified 5 January 2008

Main Sections

THERMOCALC Stuff