Practical Aspects of Mineral Thermobarometry

Thermobarometer Calibration

The aim of this section will be to cover briefly such topics such as

Conditions of heterogeneous equilibrium
The equilibrium constant and its P-T dependence.
A simple geothermometer: garnet-biotite Fe-Mg exchange
A geobarometer based on a net-transfer reaction with large volume change
How thermometers and barometers are calibrated
Self-consistent thermodynamic data sets and their application to thermobarometry

You can read about these subjects in Spear 1993, chapter 8, pp. 241-244, and chapter 15, pp. 511-537.

Here's some provisional content, from lecture course handouts:

Condition for heterogeneous equilibrium

[Spear, p. 511]. A balanced chemical reaction such as Grs + 2 Ky + Qtz = 3 An could also be written in the form 3 An - Grs - 2 Ky - Qtz = 0, reminding us that the mass of each system component is conserved, and that reaction coefficients can be positive ("products") or negative ("reactants"). At equilibrium, a similar relationship holds among the chemical potentials of phase components (n are the reaction coefficients and there are m phase components):

i.e. there is no free energy difference between "reactants" and "products".

Expand the expression for heterogeneous equilibrium

[Spear, p. 512]. Adding up, for all the phase components, the expressions µ_i = µ°_i + RTln(a_i), gives us

G_R = 0 = G⁰ + RTlnK

where K is the equilibrium constant or activity product, which depends on measured phase compositions. G⁰ is the standard state free energy change - something we can calculate if we have thermochemical data. Expanding G⁰ to see what thermochemical data are needed, we get:

The subscripts and limits reflect the fact that thermochemical data are generally tabulated at 298K and 1 bar.

Linear approximation for solid-solid reactions

[Spear, p. 523]. C_P = 0. The C_P terms adjust H and S for different P and T. The heat capacities of solid phases vary sympathetically, so C_P is small, and can often be ignored, particularly over small ranges of temperature.

V constant. The volume integral brings the enthalpies from their reference pressure P⁰ (1 bar) up to the pressure of interest (usually several thousand bars). V is not a function of P or T if all solids are roughly equally compressible, so the volume integral becomes V(P - P⁰). Also, P⁰ is negligible compared to P.

This gives us the greatly simplified expression

which, for a given value of lnK, is the equation of a straight line on a P-T diagram. The curve for lnK = 0 (i.e. K = 1) is the curve for pure phases, such as might be determined in the experimental laboratory. Other values of lnK displace the curve across the P-T diagram.

Dependence of lnK on T and P

[Spear, pp. 515-6]

where S and V are calculated at the P and T of interest. Also remember the Clausius-Clapeyron equation:

; or, more strictly,

Therefore, a good geobarometer needs a large V, in order to have a large sensitivity to P and for curves of constant K to have a low slope on the P-T diagram.

Geothermometry and geobarometry using multivariant equilibria

At equilibrium

DG_P,T = DH_1,T - T.DS_T + (P-1).DV + RT.lnK = 0

K is the equilibrium constant, the product of the activities of end members in the phases of variable composition.

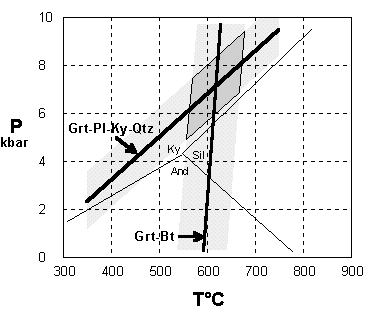
The Grt-Pl-Ky-Qtz geobarometer

Ca₃Al₂Si₃O₁₂ + 2Al₂SiO₅ + SiO₂ = 3CaAl₂Si₂O₈

grossular(Grt) + kyanite + quartz = anorthite(Pl)

Substituting thermochemical data, dividing through by the gas constant R, and expanding K gives the calibration:

7635 - 19.66T + 0.7963(P-1) + 3Tln(X_Ca,Pl/X_Ca,Grt) = 0

DV is large, because of the density difference between open feldspar and close-packed garnet and kyanite structures. The P-T slope is relatively low, making this equilibrium useful as a barometer. Uncertainty on calculated P is about ± 1.3 kbar.

The Grt-Bt Fe-Mg exchange thermometer

Fe₃Al₂Si₃O₁₂ + KMg₃AlSi₃O₁₀(OH)₂ = Mg₃Al₂Si₃O₁₂ + KFe₃AlSi₃O₁₀(OH)₂

Almandine (Grt) + Phlogopite (Bt) = Pyrope(Grt) + Annite (Bt)

DG for cation exchange is small, and the calibration here is fitted to exchange equilibrium experiments

6266 - 2.35T + 0.029(P-1) + 3TlnK_D = 0

K_D is most simply expressed as (Mg/Fe)_Grt /(Mg/Fe)_Bt

DV is very small, and cation exchange equilibria are generally insensitive to pressure. The usually-quoted uncertainty on calculated T is ± 50°.

Application

An amphibolite-facies metapelitic schist from the Eastern Alps contains garnet of composition (% end members) Alm₈₀Sps₃Prp_13.5Grs_3.5 , biotite with Mg/Fe = 0.91, and plagioclase An15 , together in apparent equilibrium with kyanite and quartz. From these data we calculate the two P-T curves. Their intersection (with a surrounding box of uncertainty) gives us an estimate of the conditions of equilibration.

Self-consistent data-sets

[ Material to incorporate from Petrology course lecture slides ]

^ Top

This page last modified 12 October 2004

Main Sections

THERMOCALC Stuff