Practical Aspects of Mineral Thermobarometry

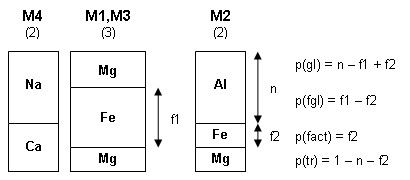
Activity coding for Na-amphibole

Four-component NCFMASH amphibole

This provisional coding for sodic amphiboles (18 Aug 2003) expresses them in the quadrilateral gl-fgl-tr-fact. There are separate terms for the Fe content of the M1/M3 and M2 sites - it seems to work OK. The fictive Ca-amphibole end members are given relatively large DQF increments, following Carson et al., 1999, Journal of Metamorphic Geology, 17, 9-24. These restrict the Ca content of sodic amphibole, and could be adjusted to match well-characterised natural compositions.

nam 4
 
    n(nam) 0.8     % Na/(Na+Ca)
    f1(nam) 0.3    % XFe in M13
    f2(nam) 0.05   % XFe in M2

    p(gl)   1  1    0 3  1  n -1 f1  1 f2      % n-f1+f2
    p(fgl)  1  1    0 2  1 f1 -1 f2            % f1-f2
    p(tr)   1  1    1 2 -1  n -1 f2            % 1-n-f2
    p(fact) 1  1    0 1  1 f2
    
ideal

6   
    x(Na,M4)    1 1   0 1  1 n          % n
    x(Ca,M4)    1 1   1 1 -1 n          % 1-n        
    x(Mg,M13)   1 1   1 1 -1 f1         % 1-f1
    x(Fe,M13)   1 1   0 1  1 f1         % f1
    x(Mg,M2)    1 1   1 2 -1 n  -1 f2   % 1-n-f2
    x(Fe,M2)    1 1   0 1  1 f2         % f2
    % note that x(Al,M2) = x(Na,M4) = n

% Ideal Mixing activities

    gl  1 2  x(Na,M4) 4  x(Mg,M13) 3
    fgl 1 2  x(Na,M4) 4  x(Fe,M13) 3 
    tr  1 3  x(Ca,M4) 2  x(Mg,M13) 3  x(Mg,M2) 2
        DQF 46  0  0                  % From Carson 99
    fact 1 3  x(Ca,M4) 2  x(Fe,M13) 3  x(Fe,M2) 2
        DQF 46  0  0
    % DQF increments should serve to restrict the Ca content of gl-fgl s.s.
    % could adjust these to correspond to observed/experimental compositions

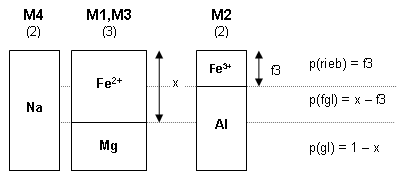
Glaucophane-crossite-riebeckite series (NFMASHO)

This treatment is adapted from the documentation for the AX program, and has been tested on blue amphiboles in garnet blueschists and eclogites from As Sifah, Oman, whose estimated Fe³⁺ contents range from about 20 to 50%. A DQF adjustment to the ferroglaucophane end member of about -14 kJ is required to bring the calculated Fe/Mg ratios into concordance with those of coexisting garnet and pyroxene in a range of natural samples. Adjusting the entropy of fgl (incorporating T dependence in the DQF factor, while holding the total correction at around -14 kJ in the range 500-550°C) also removes an abnormal T dependence from the K_D for these Fe-Mg partitions. Furthermore, the ferric iron contents of natural crossites in relation to coexisting epidote and clinopyroxene are matched when an adjustment of +10 kJ is made to the (fictive) riebeckite end member.

I don't know the basis for determining the values of the interaction parameters; I just took these from the AX documentation. I'm a little unhappy about using symmetric formalism in a model where p(fgl) can readily take on negative values; but the calculated results are not very sensitive to the W's, and one could equally use an ideal mixing model.

nam 3

  x(nam)  0.3    % = Fe/(Fe+Mg)
  f3(nam) 0.45   % = Fe3/(Al+Fe3)

  p(gl)    1 1   1  1 -1  x
  p(fgl)   1 1   0  2  1  x -1  f3
  p(rieb)  1 1   0  1  1  f3 

  sf
  W(gl,fgl)   10  0  0
  W(gl,rieb)  10  0  0
  W(fgl,rieb)  0  0  0

  4
  X(Mg,M13)  1 1   1 1 -1 x
  X(Fe,M13)  1 1   0 1  1 x
  X(Al,M2)   1 1   1 1 -1 f3
  X(F3,M2)   1 1   0 1  1 f3

  gl    1 2    X(Mg,M13) 3   X(Al,M2) 2
  fgl   1 2    X(Fe,M13) 3   X(Al,M2) 2
        DQF -4.4 -0.012 0    % to improve Fe-Mg distrib and overall stability
  rieb  1 2    X(Fe,M13) 3   X(F3,M2) 2
        DQF   10  0  0       % otherwise too much rieb

^ Top

This page last modified 1 November 2004

Main Sections

THERMOCALC Stuff

Practical Aspects of Mineral Thermobarometry

Activity coding for Na-amphibole

Four-component NCFMASH amphibole

Glaucophane-crossite-riebeckite series (NFMASHO)